Time‐periodic and stationary solutions to the compressible Hall‐MHD system

程明，吉林大学, jlumcheng@hotmail.com

Abstract: In the present paper, we are concerned with the 3‐D compressible Hall‐magnetohydrodynamic system with a time‐periodic external force in a periodic domain. We obtain the existence of a strong time‐periodic solution under some smallness and symmetry assumptions by a new approach. First, we prove the existence of the time‐ periodic solution to a linearized system by Tychonoff fi xed point theorem combining with the energy method and the decay estimates. From the details of the proof, it shows that the initial data of the time‐periodic solution to the linearized system lies in some convex hull. Second, we construct a set‐value function such that the fixed point of this function is the time‐periodic solution of the compressible Hallmagnetohydrodynamic system. The existence of the fixed point is obtained by the Kakutani fixed point theorem. Moreover, we establish the uniqueness of the time‐ periodic solution and the existence of the stationary solution.

Some studies on the relaxation models in hydrodynamics

胡玉玺，中国矿业大学, hu‐yuxi@163.com

Abstract: The compressible Navier‐Stokes equations are to describe the dynamics of compressible fluid. The system are composed of mass equation, momentum equation and energy equation. Mathematically, the system are underdetermined and one need to give constitutive equations to close the system. Newtonian law in describing the relation of stress tensor and velocity, and Fourier law in describing the heat conductivity are the two main constitutive relations in fluid dynamics. In this talk, we shall investigate some other constitutive relations, namely, Cattaneo's law for heat conductivity and Maxwell's law for the stress tensor and velocity, which can be considered as some kind of relaxation of the classical system. The importance of these two constitutive relations are demonstrated by many physicists and biologists recently, in the field such as skin burns, nano fluids , biological materials, nanoscale mechanical devices vibrating in simple fluid, etc. However, the corresponding mathematical results are still very few. We shall present some related mathematical results and give some new results.

On stochastic primitive equations of the large‐scale ocean

黄代文教授，北京应用与计算数学研究所, huang_daiwen@iapcm.ac.cn

Abstract: Tn this talk, we give some results on stochastic primitive equations of the large‐scale ocean. Firstly, we recall the global well‐posedness and long‐time dynamics for the viscous primitive equations describing the large‐scale oceanic motion with white noise. Secondly, we introduce some results on ergodicity of the stochastic primitive equations driven by degenerate noise such.

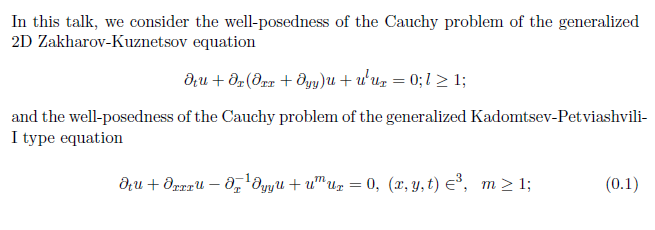
Well‐posedness for the 2D generalized Zakharov‐Kuznetsov equation and generalized Kadomtsev‐Petviashvili‐I type equation

霍朝辉，中科院数学与系统科学研究院， zhhuo@amss.ac.cn

Abstract:

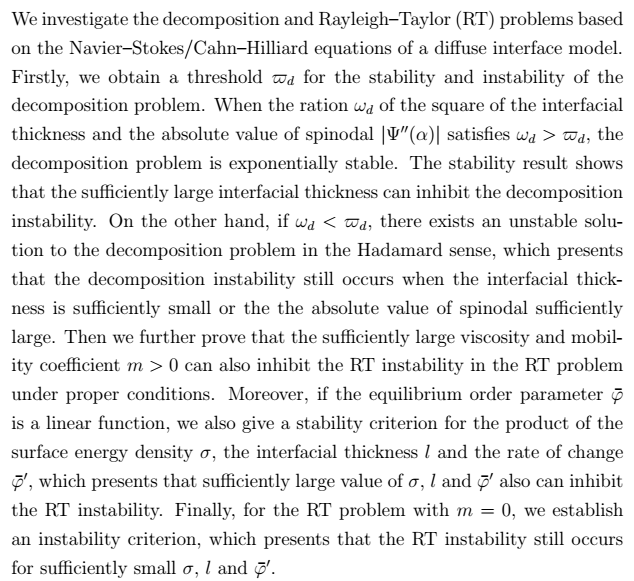
Some Stablity and Instablity Results in a Diffuse Interface Model

江飞，福州大学， jiangfei0591@163.com

Abstract:

Singular limits of the equations of compressible ideal magneto‐hydrodynamics in a domain with boundaries

琚强昌，北京应用与计算数学研究所， ju_qiangchang@iapcm.ac.cn

Abstract: Singular limits of the equations of compressible ideal magneto‐hydrodynamics with physical boundary conditions are considered. The uniform existence of classical solutions with respect to both the reciprocals of Mach and Alfv'{e}n numbers are established by energy methods. Under appropriate conditions on the initial data, convergence of the solutions of the original system is proved as the two large parameters tend to infinity with the limiting solutions satisfying the two‐dimensional incompressible flow. This is a recent joint work with Prof. Steve Schochet and Dr. Xin Xu.

Asymptotic limit of the compressible Navier‐Stokes‐Fourier‐P1 approximation model

栗付才，南京大学， fli@nju.edu.cn

Abstract: TIn this talk I shall discuss our recent results on the the asymptotic limit of the compressible Navier‐Stokes‐Fourier‐P1 approximation model arising in radiation hydrodynamics. We shall also review some related results on the model..

Quasineutral limit of the two‐fluid Euler‐Poisson system in a bounded domain

黎勇，北京工业大学， yli@bjut.edu.cn

Abstract: The quasineutral limit of the two‐fluid Euler‐Poisson system (one for ions and another for electrons) in a bounded domain of $mathbb{R}^3$ is rigorously proved by investigating the existence and the stability of boundary layers. The limit is one‐fluid compressible Euler equations when the leading profiles of the initial velocities for two particles are assumed to be same.The non‐penetration boundary condition for velocities and Dirichlet boundary condition for electric potential are considered.

On the Inviscid Limit of the 3D Navier‐Stokes equation

肖跃龙，湘潭大学， xyl@xtu.edu.cn

Abstract:

On the Free Surface Motion of Highly Subsonic Heat‐conducting Inviscid Flows

曾惠慧，清华大学丘成桐数学科学中心， hhzeng@mail.tsinghua.edu.cn

Abstract: In this talk, I will present a recent result joint with Tao Luo on the free surface problem of a highly subsonic heat‐conducting inviscid flow. Adopting a geometric approach developed by Christodoulou and Lindblad in the study of the free surface problem of incompressible inviscid flows, we give the a priori estimates of Sobolev norms in 2‐D and 3‐D under the Taylor sign condition by identifying a suitable higher order energy functional. The estimates for some geometric quantities such as the second fundamental form and the injectivity radius of the normal exponential map of the free surface are also given. I will discuss the issues of the strong coupling of large variation of temperature, heat‐conduction, compressibility of fluids and the evolution of free surface, loss of symmetries of equations, and loss of derivatives in closing the argument which is a key feature compared with Christodoulou and Lindblad's work.